[085]SW VSYNC模型更新与校准

王小二

发布于 2023-11-04 08:42:01

4710

发布于 2023-11-04 08:42:01

背景

对于Vsync信号的相关领域中，一直有一座大山我一直没有翻跃，那就是SW VSYNC模型更新与校准。经过认真阅读努比亚技术团队的文章SurfaceFlinger模块-VSYNC研究，我终于翻跃了这座大山。本文是针对SW VSYNC模型更新与校准这部分的理解和补充，建议先看努比亚的文章再看我这个文章。

1.根据采样的时间戳更新模型

对应SurfaceFlinger模块-VSYNC研究文章中3.2 1 addVsyncTimestamp章节。通过着6个样本，计算出x的因变量集合 ordinals，和y的自变量集合vsyncTS。通过6个样本把这两个集合的数据都计算出来，然后通过上面的方程式把回归系数和截距都计算出来，这块的回归系数就是Vsync的时间周期，前面我加过日志，我把这两个集合的内容可以贴出来看下，以下是90fps的vsync信息。 x的集合内容 {0，1000，2000，3000，4000，5000} ，从集合的内容是vsync的个数信息。 y的集合内容{0，11027000，22053000，33080000，44106000，55132000}，从代码中了解是硬件vsync时间戳的递增值，

看了有点蒙圈，假设传入连续的六个时间戳，t是为任意值。

{t，t + 11027000，t + 22053000，t + 33080000，t + 44106000，t + 55132000}

我们得到的其实是集合映射关系是M~T，m代表第m个vsync信号，t就是第m个信号时间戳，单位纳秒，也就是第m个vsync时间戳为t纳秒，第m+1个vsync时间戳为t + 11027000纳秒

M {m, m+1, m+2, m+3, m+4, m+5} ~ T {t，t + 11027000，t + 22053000，t + 33080000，t + 44106000，t + 55132000}

这个M-T映射关系和上面努比亚文章中的x-y集合映射有什么关系呢？

x{0，1000，2000，3000，4000，5000} ~ y {0，11027000，22053000，33080000，44106000，55132000}

我们看代码

// normalizing to the oldest timestamp cuts down on error in calculating the intercept.
auto const oldest_ts = *std::min_element(mTimestamps.begin(), mTimestamps.end());
vsyncTS[i] = mTimestamps[i] - oldest_ts;

oldest_ts 就是T的集合中时间戳最小值，也就是t。每个时间戳在减去t，那不就得到了y集合。

y {0，11027000，22053000，33080000，44106000，55132000}

currentPeriod 在90hz屏幕下固定值为11111111，vsyncTS[i] 就是y集合中数字，其中kScalingFactor 放大因子为1000，根据以下公式，可以得到x的集合

static constexpr int64_t kScalingFactor = 1000;
ordinals[i] = ((vsyncTS[i] + (currentPeriod / 2)) / currentPeriod) * kScalingFactor;

所以我们根据传入的六个连续vsync时间戳得到了努比亚文章中所描述的X和Y集合映射。

{t，t + 11027000，t + 22053000，t + 33080000，t + 44106000，t + 55132000}
得到了
x {0，1000，2000，3000，4000，5000}  ~ y {0，11027000，22053000，33080000，44106000，55132000}

假设y=bx + a是拟合x~y映射关系的函数，用以下公式来就先求中b的值 b = slope = top/bottom。

     //         Sigma_i( (X_i - mean(X)) * (Y_i - mean(Y) )      -----> top
     // slope = -------------------------------------------
     //         Sigma_i ( X_i - mean(X) ) ^ 2                  -----> bottom

假设x=1000x'，如何求y=b'x' + a'的函数曲线？

直接将1000x'带入y=bx + a中即可得y=b*1000x' + a 所以b' = 1000b, a' = a , 其中b'也就是下面这段代码中anticipatedPeriod = 1000 * slope = 1000 * top/bottom。

nsecs_t const anticipatedPeriod = top * kScalingFactor / bottom;//kScalingFactor  = 1000

因为a' = a，所以只要按照以下公式求得a，也就是intercept 就好了

     //
     // intercept = mean(Y) - slope * mean(X)
     //

但是由于anticipatedPeriod = 1000 * slope，所以下面需要除以kScalingFactor（1000）才能得到正确的intercept，也就是a'。

nsecs_t const intercept = meanTS - (anticipatedPeriod * meanOrdinal / kScalingFactor);

小结

通过转化，将集合T转化成了x-y的映射关系

T {t，t + 11027000，t + 22053000，t + 33080000，t + 44106000，t + 55132000}
经过转化得到
x{0，1000，2000，3000，4000，5000} ~ y {0，11027000，22053000，33080000，44106000，55132000}

根据x-y的映射关系，算出了y=b'x' + a'中b'和a'

b' = anticipatedPeriod
a' = intercept

因为x=1000x'，所以 y=b'x' + a'描述以下x'-y的映射关系的直线，所以努比亚文章中描述有点不准确。

x' {0，1，2，3，4，5}  ~ y {0，11027000，22053000，33080000，44106000，55132000}

为什么要加入1000的缩放因子，应该是为了提高计算的精准度。

二、已知上一次的vsync的时间戳求next vsync的时间戳

目前我们已经通过前面得到了一个函数y=b'x' + a'来表示以下集合的映射关系,其中b' = slope，a' = intercept

x' {0，1，2，3，4，5}  ~ y {0，11027000，22053000，33080000，44106000，55132000}

根据模型获得slope斜率，以及截距intercept，用图表示如下。

auto const [slope, intercept] = getVSyncPredictionModelLocked();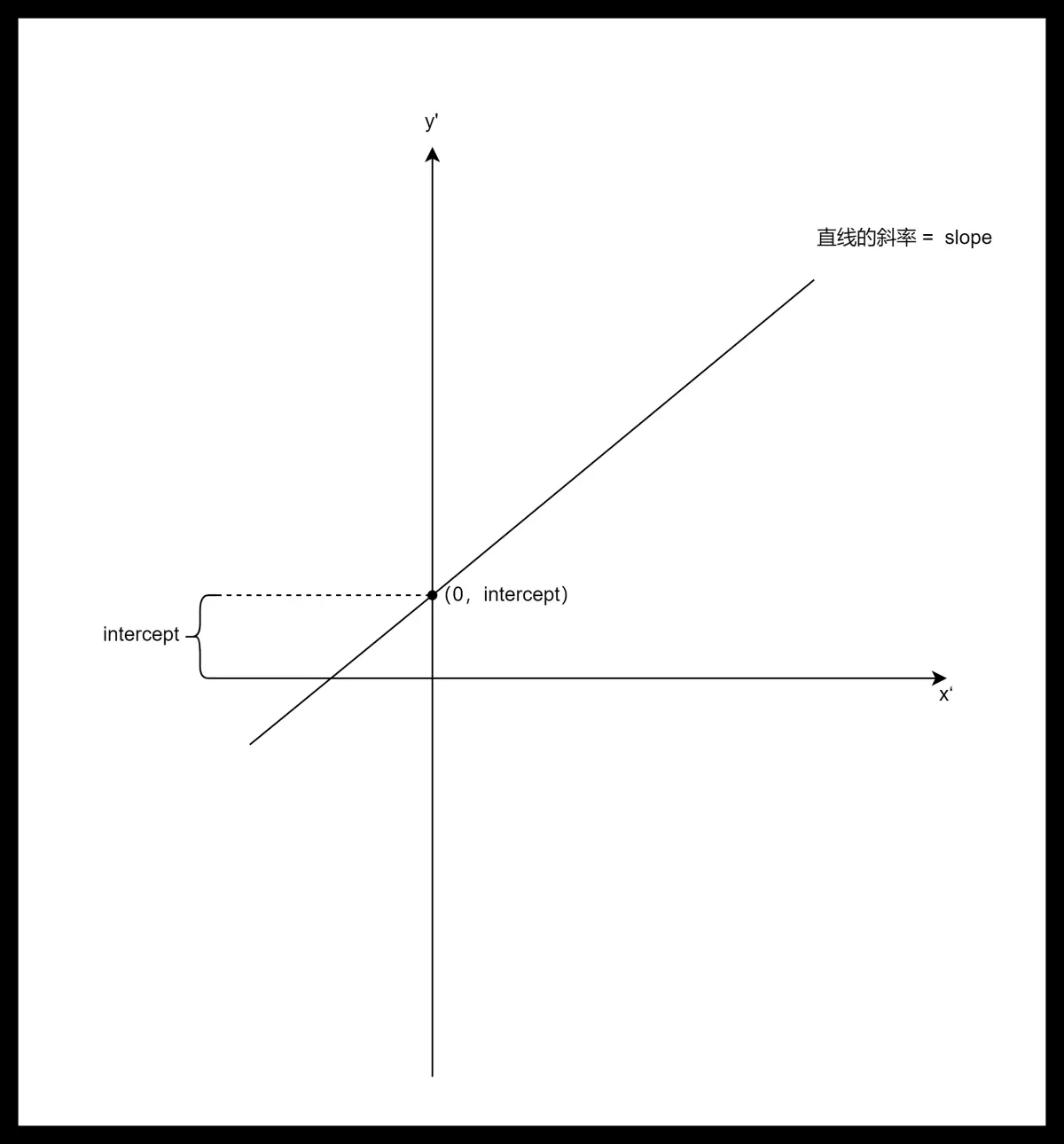

假设我们上一次的vsync时间戳为Time1，如果求得下一个vsync的时间戳Time2呢？

努比亚团队是这样子描述的，有点不是很清楚。如果mTimestamps的集合不为空，通过这个集合的数据和传入的发射时间，算出一次线程回归方式的因变量x值，然后根据回归系数和截距，用方程式计算出自变量y值，而y值，也就是代码中的prediction，作为下一次vsync发射的时间。

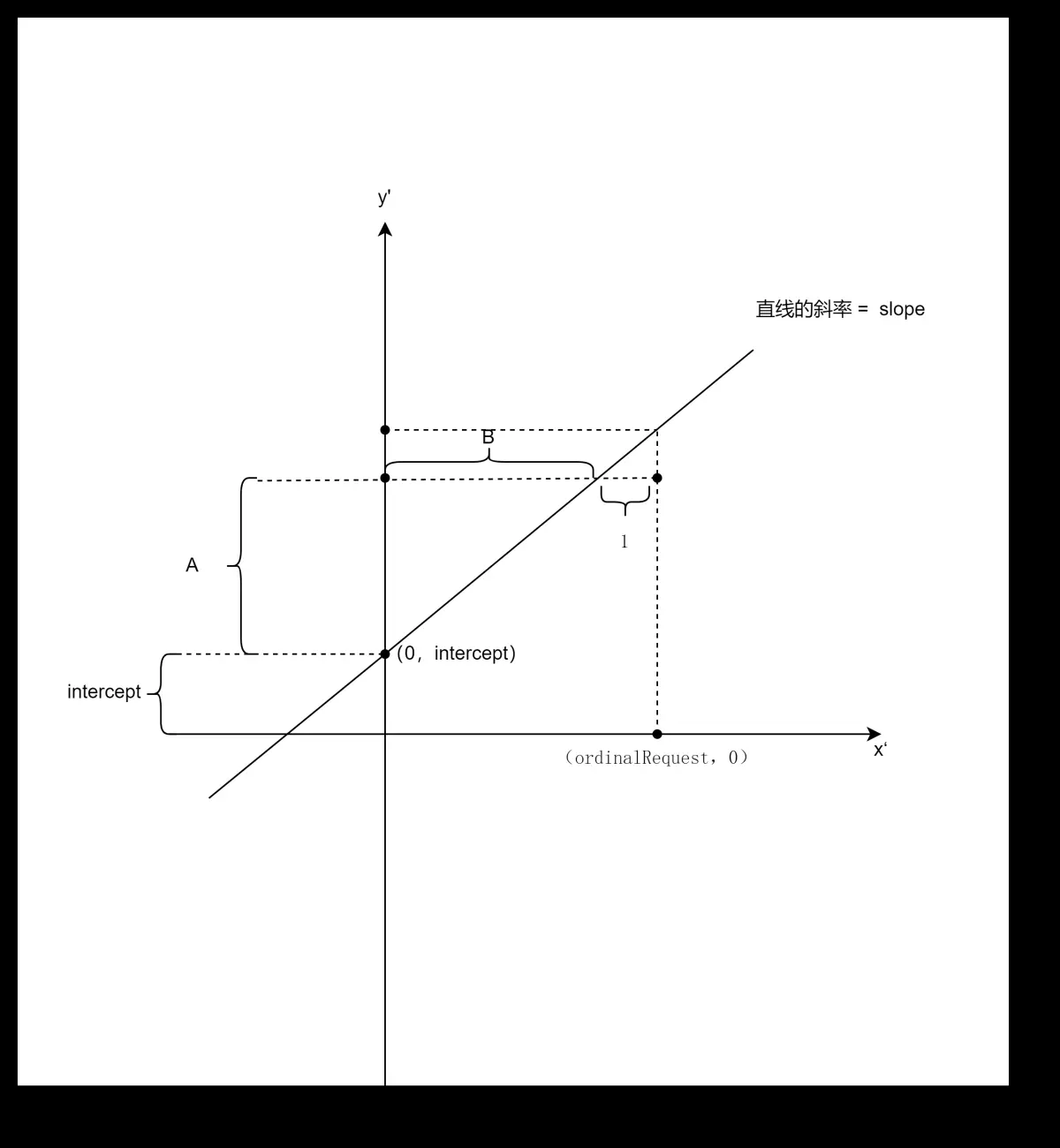
以下代码描述了这个计算过程

    auto const oldest = *std::min_element(mTimestamps.begin(), mTimestamps.end());
    // See b/145667109, the ordinal calculation must take into account the intercept.
    auto const zeroPoint = oldest + intercept;
    auto const ordinalRequest = (timePoint - zeroPoint + slope) / slope;
    auto const prediction = (ordinalRequest * slope) + intercept + oldest;

用图来简单表示一下，可以好好思考一下，初中数学知识应该就就可以看得懂上述的计算方式。其中A=timePoint - zeroPoint